Shanghai University
Article Information
- Xie-ping DING . 2014.
- Behavior of solution set for bilevel generalized mixed equilibrium problems in topological vector spaces
- Appl. Math. Mech. -Engl. Ed., 35(7): 925-934
- http://dx.doi.org/10.1007/s10483-014-1832-9
Article History
- Received 2013-5-27;
- in final form 2013-9-22
The bilevel problem and mathematical program problem with equilibrium constraint repre- sent important classes of optimization problems which have been wildly investigated in a large number of articles and books. Recently,Moudafi[1] studied a class of bilevel monotone equilib- rium problems in Hilbert spaces and suggested an iterative algorithm to compute approximate solutions of the problem and proved the weak convergence of the iterative sequence generated by the algorithm. Since then,many authors have further introduced and studied the bilevel mixed equilibrium problem (BMEP),the bilevel pseudomonotone equilibrium problem,and the bilevel generalized mixed equilibrium problems (BGMEP) in finite dimensional spaces and Banach spaces,respectively. For example,see [2, 3, 4, 5, 6, 7, 8, 9, 10, 11] and the references therein.
In this paper,we introduce and study a new class of BGMEP involving set-valued mappings in topological vector spaces. By using a minimax inequality due to Ding and Tan[12],some existence theorems of solutions for the generalized mixed equilibrium problems (GMEP) and BGMEP are proved under quite mild conditions. The behavior of the solution set for the problems are also discussed. These results are new and generalize some results in this field.
Let E be a Hausdorff topological vector space with dual space E*,and let h·,·i denote the duality pairing between E* and E. Let C be a closed convex subset of E,and C(E*) denotes the family of all compact subsets of E*. Let F,H : E*×C×C → R and Φ, Ψ,φ,ψ: C×C → R be real-valued functions,N,M : E* × E* → E* be single-valued mappings,and A,T,Q,S :C → C(E*) be set-valued mappings. We consider the following BGMEP involving bifunctions and set-valued mappings:
where SΦ,φF,N,A,T is the solution set of the following GMEP involving bifunctions and set-valued mappings:Case I If ≡ Φ ≡ 0,then the BGMEP (1)-(2) reduce to the following BGMEP involving bifunctions and set-valued mappings:
where SΦ,φF,N,A,T is the solution set of the following GMEP involving bifunctions and set-valued mappings:The BGMEP (3)-(4) were introduced and studied in Banach spaces by Ding et al.[6].
Case II If for given !,b! ∈ E* and η,bη : C × C → E,let F(N(u,v),x,y) = hN(u,v) −
!,η(y,x)i and  ,then
the BGMEP (1)-(2) reduce to the following bilevel generalized mixed variational-like inequality
problem (BGMVIP): for given !,! ∈ B*,
,then
the BGMEP (1)-(2) reduce to the following bilevel generalized mixed variational-like inequality
problem (BGMVIP): for given !,! ∈ B*,
The BGMVIP (5)-(6) were introduced and studied by Ding[4, 5] in reflexive Banach spaces, which include the BMEP (1.3) introduced and studied by Chen et al.[11] as special cases.
For suitable choice of Φ, ,H,F,M,N,A,T ,Q,S,,and ',it is easy to see that the BGMEP (1)-(2) include a lot of BGMEP,BGMVIP,GMEP,and GMVIP studied by many authors as special cases. For example,see [1 24] and the references therein.
2 PreliminariesDefinition 2.1 Let X and Y be topological spaces. A set-valued T : X → 2Y is said to be
(i) upper semicontinuous if for each closed set D ⊆ Y ,the set T+1(D) = {x ∈ X : T (x)∩D ≠∅ } is closed in X;
(ii) lower semicontinuous if for each open set D ⊆ Y ,the set T+1(D) is open in X;
(iii) continuous if it is both upper and lower semicontinuous.
Definition 2.2 Let C be a closed convex subset of a topological vector space E. The bifunction Φ : C × C → R is said to be monotone if

Definition 2.3 Let C be a closed convex subset of a topological vector space E. Let N : E* × E* → E* be a single-valued mapping,A,T : C → C(E*) be set-valued mappings,and F : E* × C × C → R be a real-valued function. F is said to be monotone with respect to N,A, and T if

Remark 2.1 For the example of Definition 2.3,the reader may consult Remark 2.2 in Ref. [6].
Definition 2.4 Let E be a topological vector space. The bifunction ' : E ×E → R is said to be skew-symmetric if

For the properties and applications of the skew-symmetric bifunction,the reader may consult Antipin[25].
Lemma 2.1[26] Let X and Y be topological spaces and T : X → 2Y be a set-valued mapping.
(i) If X is compact and T is upper semicontinuous with compact values,then T (X) is compact.
(ii) If Y is compact and T is closed,then T is upper semicontinuous.
(iii) If T is upper semicontinuous with closed values,then T is closed.
The following results are Theorem 7.3.11 and Theorem 7.3.14 of Klein and Thompson[27].
Lemma 2.2 Let X,Y,and Z be topological spaces. Let F : X → 2Y and G : Y → 2Z be set-valued mappings.
(i) If F and G are upper semicontinuous,then G ◦ F : X → 2Z is upper semicontinuous.
(ii) If F and G are lower semicontinuous,then G ◦ F : X → 2Z is lower semicontinuous.
Lemma 2.3 Let I be an index set. Let X and Yi,i ∈ I be all topological spaces. For each
i ∈ I,let Fi : X → 2Yi be set-valued mappings. Let F =  be defined by F(x) =
be defined by F(x) =  (x).
If each Fi is upper continuous with compact values,then F is also upper semicontinuous with
compact values with respect to the product topology on
(x).
If each Fi is upper continuous with compact values,then F is also upper semicontinuous with
compact values with respect to the product topology on  .
.
Lemma 2.4[28] Let X and Y be two topological spaces. Let F : X×Y → R be a bifunction
and S : X → 2Y be a set-valued mapping with nonempty values and let m(x) =  .
.
(i) If F and S are both lower semicontinuous,then m is also lower semicontinuous.
(ii) If F is upper semicontinuous and S is upper semicontinuous with compact values,then m is also upper semicontinuous.
Lemma 2.5 Let C be a nonempty convex subset of a topological vector space E. Let N : E* × E* → E* and A,T : C → C(E*) be upper continuous. Let F : E* × C × C → R such that for each fixed y ∈ C,(u,x) → F(u,x,y) is upper continuous. Then,for each (x,y) ∈ C×C, there exists (u,v) ∈ A(x) × T (x) such that

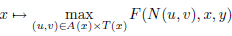 is upper semicontinuous.
is upper semicontinuous.
Proof Since N : E* × E* → E* is upper semicontinuous and for each y ∈ C,(u,x) →
F(u,x,y) is upper semicontinuous,it follows from Lemma 2.2 that for each y ∈ C,the mapping
((u,v),x) 7→ F(N(u,v),x,y) is also upper semicontinuous. By Lemma 2.3,the mapping A×T :
C → C(E*)×C(E*) defined by (A×T )(x) = A(x)×T (x) is upper semicontinuous with compact
values. Since for each x ∈ C,A(x) × T (x) is compact in E* × E*,it follows that there exists
(u,v) ∈ A(x)×T (x) such that  . It follows from
Lemma 2.4 that the mapping
. It follows from
Lemma 2.4 that the mapping 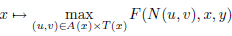 is upper semicontinuous.
is upper semicontinuous.
The following result is a special case of Theorem 1 of Ding and Tan[12] (Also see Lemma 2.7 of Ding et al.[6]).
Lemma 2.6 Let C be a nonempty convex subset of a topological vector space and let f : C × C → [−∞,+∞] such that
(i) f(x,x) > 0 for each x ∈ C;
(ii) for each y ∈ C,x ↔ f(x,y) is upper semicontinuous on each nonempty compact subset of C;
(iii) for each x ∈ C,y ↔ f(x,y) is convex;
(iv) there exists a nonempty compact subset K of C and y ∈ K such that f(x,y) < 0, ∀x ∈ C \ K. Then,there exists a point bx ∈ K such that f(bx,y) > 0 for all y ∈ C. 3 Behavior of solution set of GMEP (2)Theorem 3.1 Let C be a closed convex subset of topological vector space E. Let F : E* × C × C → R,N : E* × E* → E*,A,T : C → C(E*),Φ : C × C → R,and φ : B × B → R satisfy the following conditions:
(i) ;
;
(ii) Φ is monotone and F is monotone with respect to N,A,and T ;
(iii) Φ is upper semicontinuous in the first argument and is convex and lower semicontinuous in the second argument;
(iv) for each y ∈ C,((u,v),x) ↔ F(N(u,v),x,y) is upper semicontinuous and for each z ∈ C and (u,v) ∈ A(z) × T (z),y ↔ F(N(u,v),z,y) is convex and lower semicontinuous;
(v) A and T are both upper semicontinuous;
(vi) ' is skew symmetric and continuous,and ' is convex in the first argument.
Then,there exists x ∈ C such that
if and only if Furthermore,the solution set SΦ,φF,N,A,T of the GMEP (2) is a closed convex subset of C.Proof For any y ∈ C,define two mappings G and P as follows:

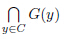 =
=
 . Since Φ is monotone,we have
. Since Φ is monotone,we have



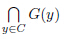 ⊆
⊆  . Conversely,if there
exists x ∈
. Conversely,if there
exists x ∈  ,but x ≠
,but x ≠ 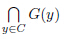 ,then we have
and there exists y ∈ C such that
It follows that
Let xt = ty + (1 − t)x = x + t(y − x),t ∈ [0, 1]. Then,xt ∈ C. It follows from (9) that
Since for each y ∈ C,((u,v),x) ↔ F(N(u,v),x,y) is upper semicontinuous and each A(xt) ×
T (xt) is compact,it follows that for each t ∈ (0,1],there exists (ut,vt) ∈ A(xt) × T (xt) such
that
,then we have
and there exists y ∈ C such that
It follows that
Let xt = ty + (1 − t)x = x + t(y − x),t ∈ [0, 1]. Then,xt ∈ C. It follows from (9) that
Since for each y ∈ C,((u,v),x) ↔ F(N(u,v),x,y) is upper semicontinuous and each A(xt) ×
T (xt) is compact,it follows that for each t ∈ (0,1],there exists (ut,vt) ∈ A(xt) × T (xt) such
that
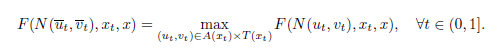 By (12),we have
Let Ω = {xt}t∈[0 1]. Then,
is compact. Since A and T are both upper semicontinuous with
compact values,it follows from Lemma 2.1 that A(Ω) × T (Ω)is compact in E* × E*. Noting
(ut,vt)t2[0, 1] ⊂ A(Ω)× T (Ω)and lim
t!0
xt → x,without loss of generality,we can assume that
there exists (u,v) ∈ E*×E* such that (ut,vt) → (u,v) and (u,v) ∈ A(x)×T (x). Since for each
y ∈ C,x ↔ Φ(x,y) and ((u,v),x) ↔ F(N(u,v),x,y) are upper semicontinuous,x ↔ −Φ(x,y)
and ((u,v),x) ↔ −F(N(u,v),x,y) are lower semicontinuous. As ' is continuous,by (13),we
have that
By (12),we have
Let Ω = {xt}t∈[0 1]. Then,
is compact. Since A and T are both upper semicontinuous with
compact values,it follows from Lemma 2.1 that A(Ω) × T (Ω)is compact in E* × E*. Noting
(ut,vt)t2[0, 1] ⊂ A(Ω)× T (Ω)and lim
t!0
xt → x,without loss of generality,we can assume that
there exists (u,v) ∈ E*×E* such that (ut,vt) → (u,v) and (u,v) ∈ A(x)×T (x). Since for each
y ∈ C,x ↔ Φ(x,y) and ((u,v),x) ↔ F(N(u,v),x,y) are upper semicontinuous,x ↔ −Φ(x,y)
and ((u,v),x) ↔ −F(N(u,v),x,y) are lower semicontinuous. As ' is continuous,by (13),we
have that


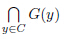 =
=  . It is easy to see that SΦ,φF,N,A,T =
. It is easy to see that SΦ,φF,N,A,T = 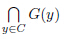 . Therefore,
SΦ,φF,N,A,T =
. Therefore,
SΦ,φF,N,A,T = 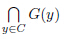 =
=  is the solution set of GMEP (2). By the definition of P,for
each y ∈ C,we have
Since for each y ∈ C and (w,z) ∈ A(y) × T (y),x ↔ F(N(w,z),y,x),x ↔ Φ(y,x),and
x ↔ '(x,y) are all convex and lower semicontinuous,it follows that for each y ∈ C,the set
{x ∈ C : F(N(w,z),y,x) + '(x,y) 6 '(y,y)} is closed and convex. Hence,P(y) and
is the solution set of GMEP (2). By the definition of P,for
each y ∈ C,we have
Since for each y ∈ C and (w,z) ∈ A(y) × T (y),x ↔ F(N(w,z),y,x),x ↔ Φ(y,x),and
x ↔ '(x,y) are all convex and lower semicontinuous,it follows that for each y ∈ C,the set
{x ∈ C : F(N(w,z),y,x) + '(x,y) 6 '(y,y)} is closed and convex. Hence,P(y) and  are both closed convex subsets of C. If the set
are both closed convex subsets of C. If the set 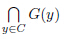 =
=  is nonempty,then there
exists bx ∈ C such that
Since for each y ∈ C,((u,v),bx) ↔ F(N(u,v),bx,y) is upper semicontinuous and A(bx)× T (bx) is
compact,it follows that there exists (bu,bv) ∈ A(bx) × T (bx) such that
It follows from (18) and (19) that there exist bx ∈ C and (bu,bv) ∈ A(bx) × T (bx) such that
is nonempty,then there
exists bx ∈ C such that
Since for each y ∈ C,((u,v),bx) ↔ F(N(u,v),bx,y) is upper semicontinuous and A(bx)× T (bx) is
compact,it follows that there exists (bu,bv) ∈ A(bx) × T (bx) such that
It follows from (18) and (19) that there exist bx ∈ C and (bu,bv) ∈ A(bx) × T (bx) such that

Remark 3.1 Theorem 3.1 improves and generalizes the corresponding results in Refs. [2, 3, 4, 5, 6, 7] to topological vector spaces.
Theorem 3.2 Let C be a closed convex subset of topological vector space E and K be a compact subset of E with C ∩ K 6= ?. Let F : E* × C × C → R and Φ,' : E × E → R be real-valued functions. Let N : E* ×E* → E* be single-valued mappings and A,T : C → C(E*) be set-valued mappings. Suppose that the following conditions are satisfied:
(i) Φ(x,x) + F(N(u,v),x,x) > 0,∀x ∈ C,(u,v) ∈ A(x) × T (x);
(ii) Φ is monotone and F is monotone with respect to N,A,and T ,and for each y ∈ C, ((u,v),x) ↔ F(N(u,v),x,y) is upper semicontinuous;
(iii) Φ is upper semicontinuous in the first argument and is convex and lower semicontinuous in the second argument and for each x ∈ C and (u,v) ∈ A(x) × T (x),y ↔ F(N(u,v),x,y) is convex;
(iv) A and T are both upper semicontinuous;
(v)φ is skew symmetric and continuous,and ' is convex in first argument;
(vi) there exists y ∈ C ∩ K such that


Proof Define a bifunction f : C × C → R as follows:


Theorem 4.1 Let C be a closed convex subset of topological vector space E and K be a compact subset of E with C ∩ K 6= ?. Let F,H : E* × C × C → R,Φ, : C × C → R,and φ,: E×E → R be real-valued functions. Let N,M : E*×E* → E* be single-valued mappings and A,T,Q,S : C → C(E*) be set-valued mappings. Suppose that the following conditions are satisfied:
(i) Φ,F,N,A,T,and ' satisfy all the conditions of Theorem 3.2;
(ii) (x,x) + H(M(u,v),x,x) > 0,∀x ∈ C,(u,v) ∈ Q(x) × S(x);
(iii) is monotone and H is monotone with respect to M,Q,and S,and for each y ∈ C, ((u,v),x) ↔ H(M(u,v),x,y) is upper semicontinuous;
(iv) is upper semicontinuous in the first argument and is convex and lower semicontinuous in the second argument and for each x ∈ C and (u,v) ∈ Q(x) × S(x),y ↔ H(M(u,v),x,y) is convex;
(v) Q and S are both upper semicontinuous;
(vi) is skew symmetric and continuous,and is convex in the first argument.
Then,the solution set of the BGMEP (1)−(2) is a nonempty compact and convex subset in C.
Proof From condition (i) and Theorem 3.2,we have that the solution set SΦ,φF,N,A,T of GMEP (2) is a nonempty compact and convex subset in C. Define a bifunction f : SΦ,φF,N,A,T × SΦ,φF,N,A,T → R as follows:

Since for each y ∈ C,((u,v),x*,y) ↔ H(M(u,v),x*,y) is upper semicontinuous and Q(x*) × S(x*) is compact in E* × E*,there exits (u*,v*) ∈ Q(x*) × S(x*) such that
It follows from (23) and (24) that there exist x* ∈ SΦ,φF,N,A,T and (u*,v*) ∈ Q(x*) × S(x*) such that
| [1] | Moudafi, A. Proximal methods for a class of bilevel monotone equilibrium problems. J. Global Optim., 47(2), 287-292 (2010) |
| [2] | Ding, X. P. Auxiliary principle and algorithm for mixed equilibrium problems and bilevel mixed equilibrium problems in Banach spaces. J. Optim. Theory Appl., 146, 347-357 (2010) |
| [3] | Ding, X. P. Existence and algorithm of solutions for mixed equilibrium problems and bilevel mixed equilibrium problems in Banach spaces. Acta Math. Sinica, 28(3), 503-516 (2012) |
| [4] | Ding, X. P. Bilevel generalized mixed equilibrium problems involving generalized mixed variational-like inequality problems in reflexive Banach spaces. Appl. Math. Mech. -Engl. Ed., 32(11), 1457-1474 (2011) DOI 10.1007/s10483-011-1515-x |
| [5] | Ding, X. P. A new class of bilevel generalized mixed equilibrium problems in Banach spaces. Acta Math. Sci., 32(4), 1571-1583 (2012) |
| [6] | Ding, X. P., Liou, Y. C., and Yao, J. C. Existence and algorithm of solutions for bilevel generalized mixed equilibrium problems in Banach spaces. J. Global Optim., 53(2), 331-346 (2012) |
| [7] | Ding, X. P. Existence and iterative algorithm of solutions for a class of bilevel generalized mixed equilibrium problems in Banach Spaces. J. Global Optim., 53(3), 525-537 (2012) |
| [8] | Chadli, O., Mahdioui, H., and Yao, J. C. Bilevel mixed equilibrium problems in Banach spaces: existence and algorithmic aspects. Numer. Algebra Cont. Optim., 1(3), 549-561 (2011) |
| [9] | Anh, P. N., Kim, J. K., and Muu, L. D. An extragradient algorithm for solving bilevel pseu- domonotone variational inequalities. J. Global Optim., 52(3), 627-639 (2012) |
| [10] | Anh, L. Q., Khanh, P. Q., and Van, D. T. M. Well-possedness under relaxed semicontinuity for bilevel equilibrium and optimization problems with equilibrium constraints. J. Optim. Theory Appl., 153, 42-59 (2012) |
| [11] | Chen, J. W., Wan, Z. P., and Cho, Y. J. The existence of solutions and well-posedness for bilevel mixed equilibrium problems in Banach spaces. Taiwanese J. Math., 17(2), 725-748 (2013) |
| [12] | Ding, X. P. and Tan, K. K. A minimax inequality with applications to existence of equilibrium point and fixed point theorems. Colloq. Math., 63, 233-247 (1992) |
| [13] | Liou, Y. C. and Yao, J. C. Bilevel decision via variational inequalities. Comput. Math. Appl., 49, 1243-1253 (2005) |
| [14] | Ding, X. P. and Liou, Y. C. Bilevel optimization problems in topological spaces. Taiwanese J. Math., 10(1), 173-179 (2006) |
| [15] | Ding, X. P. Iterative algorithm of solutions for generalized mixed implicit equilibrium-like prob- lems. Appl. Math. Comput., 162(2), 799-809 (2005) |
| [16] | Ding, X. P., Lin, Y. C., and Yao, J. C. Predictor-corrector algorithms for solving generalized mixed implicit quasi-equilibrium problems. Appl. Math. Mech. -Engl. Ed., 27(9), 1157-1164 (2006) DOI 10.1007/s10483-006-0901-1 |
| [17] | Moudafi, A. Mixed equilibrium problems: sensitivity analysis and algorithmic aspects. Comput. Math. Appl., 44, 1099-1108 (2002) |
| [18] | Kazmi, K. R. and Khan, F. A. Existence and iterative approximation of solutions of generalized mixed equilibrium problems. Comput. Math. Appl., 56, 1314-1321 (2008) |
| [19] | Ding, X. P. Existence and algorithm of solutions for nonlinear mixed quasi-variational inequalities in Banach spaces. J. Comput. Appl. Math., 157, 419-434 (2003) |
| [20] | Ding, X. P. Existence and algorithm of solutions for mixed variational-like inequalities in Banach spaces. J. Optim. Theory Appl., 127, 285-302 (2005) |
| [21] | Ding, X. P. and Yao, J. C. Existence and algorithm of solutions for mixed quasi-variational-like inclusions in Banach spaces. Comput. Math. Appl., 49, 857-869 (2005) |
| [22] | Ding, X. P., Yao, J. C., and Zeng, L. C. Existence and algorithm of solutions for generalized strongly nonlinear mixed variational-like inequalities in Banach spaces. Comput. Math. Appl., 55, 669-679 (2008) |
| [23] | Kazmi, K. R. and Khan, F. A. Auxiliary problems and algorithm for a system of generalized variational-like inequality problems. Appl. Math. Comput., 187, 789-796 (2007) |
| [24] | Xia, F. Q. and Ding, X. P. Predictor-corrector algorithms for solving generalized mixed implicit quasi-equilibrium problems. Appl. Math. Comput., 188, 173-179 (2007) |
| [25] | Antipin, A. S. Iterative gradient prediction-type methods for computing fixed-point of extremal mappings. Parametric Optimization and Related Topics IV (eds. Guddat, J., Jonden, H. T., Nizicka, F., Still, G., and Twitt, F.), Peter Lang, Frankfurt Main, 11-24 (1997) |
| [26] | Aubin, J. P. and Cellina, A. Differential Inclusions, Springer, Berlin/Heidberg/New York (1994) |
| [27] | Klein, E. and Thompson, A. C. Theory of Correspondence, John Wiley & Sons, New York (1984) |
| [28] | Lin, L. J. and Yu, Z. Y. On some equilibrium problems for multimaps. J. Comput. Appl. Math., 129, 171-183 (2001) |
 2014, Vol. 35
2014, Vol. 35


























