Applied Mathematics and Mechanics (English Edition) ›› 2025, Vol. 46 ›› Issue (1): 101-122.doi: https://doi.org/10.1007/s10483-025-3206-9
Previous Articles Next Articles
Received:2024-08-06
Revised:2024-11-22
Online:2025-01-03
Published:2025-01-06
Contact:
M. EFTEKHARI
E-mail:mo.eftekhari@uk.ac.ir
2010 MSC Number:
M. GHOLAMI, M. EFTEKHARI. Nonlinear forced vibration in a subcritical regime of a porous functionally graded pipe conveying fluid with a retaining clip. Applied Mathematics and Mechanics (English Edition), 2025, 46(1): 101-122.
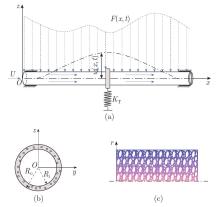
Fig. 1
(a) Coordinate system and schematic representation of the FGM porous pipe connected to an intermediary retaining clip, with clamped-clamped boundary conditions under an excitation force. (b) The cross-sectional area of the pipe. (c) Pattern for the porosity distribution (uniform distribution) (color online)"
| [1] | PAÏDOUSSIS, M. P. Pipes conveying fluid: a fertile dynamics problem. Journal of Fluids and Structures, 114, 103664 (2022) |
| [2] | IBRAHIM, R. A. Overview of mechanics of pipes conveying fluids — part I: fundamental studies. Journal of Pressure Vessel Technology, 132(3), 034001 (2010) |
| [3] | IBRAHIM, R. A. Mechanics of pipes conveying fluids — part II: applications and fluid elastic problems. Journal of Pressure Vessel Technology, 133(2), 4001270 (2011) |
| [4] | CHAI, Q., ZENG, J., MA, H., LI, K., and HAN, Q. A dynamic modeling approach for nonlinear vibration analysis of the L-type pipeline system with clamps. Chinese Journal of Aeronautics, 33(12), 3253–3265 (2020) |
| [5] | LIN, J., ZHAO, Y., ZHU, Q., HAN, S., MA, H., and HAN, Q. Nonlinear characteristic of clamp loosing in aero-engine pipeline system. IEEE Access, 9, 64076–64084 (2021) |
| [6] | ZHOU, J., CHANG, X., XIONG, Z., and LI, Y. Stability and nonlinear vibration analysis of fluid-conveying composite pipes with elastic boundary conditions. Thin-Walled Structures, 179, 109597 (2022) |
| [7] | LI, Q., LIU, W., LU, K., and YUE, Z. Nonlinear parametric vibration of a fluid-conveying pipe flexibly restrained at the ends. Acta Mechanica Solida Sinica, 33(3), 327–346 (2020) |
| [8] | MA, Y., YOU, Y., CHEN, K., and FENG, A. Analysis of vibration stability of fluid conveying pipe on the two-parameter foundation with elastic support boundary conditions. Journal of Ocean Engineering and Science, 9(6), 616–629 (2022) |
| [9] | ASKARIAN, A. R., PERMOON, M. R., ZAHEDI, M., and SHAKOURI, M. Stability analysis of viscoelastic pipes conveying fluid with different boundary conditions described by fractional Zener model. Applied Mathematical Modeling, 103, 750–763 (2022) |
| [10] | MAO, X. Y., SHU, S., FAN, X., DING, H., and CHEN, L. Q. An approximate method for pipes conveying fluid with strong boundaries. Journal of Sound and Vibration, 505, 116157 (2021) |
| [11] | WEI, S., YAN, X. FAN, X., MAO, X. Y., DING, H., and CHEN, L. Q. Vibration of fluid-conveying pipe with nonlinear supports at both ends. Applied Mathematics and Mechanics (English Edition), 43(6), 845–862 (2022) https://doi.org/10.1007/s10483-022-2857-6 |
| [12] | OYELADE, A. O., EHIGIE, J. O., OROLU, K., and OYEDIRAN, A. O. Dynamics of horizontal pipes conveying two phase flow with nonlinear boundary conditions. Mechanical Systems and Signal Processing, 217, 111520 (2024) |
| [13] | ZHANG, T., OUYANG, H., ZHANG, Y. O., and LV, B. L. Nonlinear dynamics of straight fluid-conveying pipes with general boundary conditions and additional springs and masses. Applied Mathematical Modeling, 40(17-18), 7880–7900 (2016) |
| [14] | GAO, P. X., ZHAI, J. Y., YAN, Y. Y., HAN, Q. K., QU, F. Z., and CHEN, X. H. A model reduction approach for the vibration analysis of hydraulic pipeline system in aircraft. Aerospace Science and Technology, 49, 144–153 (2016) |
| [15] | GAO, P., ZHANG, Y., LIU, X., YU, T., and WANG, J. Vibration analysis of aero parallel-pipeline systems based on a novel reduced order modeling method. Journal of Mechanical Science and Technology, 34(8), 3137–3146 (2020) |
| [16] | CHEN, W., CAO, Y., CHEN, S., GUO, X., MA, H., and WEN, B. Semi-analytical dynamic modeling of parallel pipeline considering soft nonlinearity of clamp: a simulation and experimental study. Mechanical Systems and Signal Processing, 201, 110648 (2023) |
| [17] | CAO, Y., CHEN, W., MA, H., LI, H., WANG, B., and HAN, Q. Dynamic modeling and experimental verification of clamp-pipeline system with soft nonlinearity. Nonlinear Dynamics, 111(19), 17725–17748 (2023) |
| [18] | ZHANG, L., ZHANG, T., OUYANG, H., LI, T., and ZHANG, S. Receptance-based natural frequency assignment of a real fluid-conveying pipeline system with interval uncertainty. Mechanical Systems and Signal Processing, 179, 109321 (2022) |
| [19] | ZHANG, L., ZHANG, T., OUYANG, H., LI, T., and YOU, M. Natural frequency assignment of a pipeline through structural modification in layout optimization of elastic supports. Journal of Sound and Vibration, 561, 117702 (2023) |
| [20] | ZHANG, Y., SUN, W., JI, W., and WANG, B. Hoop layouts optimization for vibration reduction of L-shaped pipeline based on substructure-analytical model and genetic algorithm. Journal of the Brazilian Society of Mechanical Sciences and Engineering, 45(5), 243 (2023) |
| [21] | LI, X., LI, W., SHI, J., LI, Q., and WANG, S. Pipelines vibration analysis and control based on clamps' locations optimization of multi-pump system. Chinese Journal of Aeronautics, 35(6), 352–366 (2022) |
| [22] | JING, J., SHAO, Z. H., MAO, X. Y., DING, H., and CHEN, L. Q. Forced resonance of a buckled beam flexibly restrained at the inner point. Engineering Structures, 303, 117444 (2024) |
| [23] | DENG, T. C., DING, H., and CHEN, L. Q. Critical velocity and supercritical natural frequencies of fluid-conveying pipes with retaining clips. International Journal of Mechanical Sciences, 222, 107254 (2022) |
| [24] | DOU, B., DING, H., MAO, X. Y., WEI, S., and CHEN, L. Q. Dynamic modeling of fluid-conveying pipes restrained by a retaining clip. Applied Mathematics and Mechanics (English Edition), 44(8), 1225–1240 (2023) https://doi.org/10.1007/s10483-023-3016-9 |
| [25] | DOU, B., DING, H., MAO, X. Y., FENG, H. R., and CHEN, L. Q. Modeling and parametric studies of retaining clips on pipes. Mechanical Systems and Signal Processing, 186, 109912 (2023) |
| [26] | DOU, B., LI, M., and DING, H. A novel retaining clip for vibration reduction of fluid-conveying pipes by piecewise constraints. Mechanical Systems and Signal Processing, 210, 111159 (2024) |
| [27] | DENG, T. C., DING, H., MAO, X. Y., and CHEN, L. Q. Natural vibration of pipes conveying high-velocity fluids with multiple distributed retaining clips. Nonlinear Dynamics, 111(20), 18819–18836 (2023) |
| [28] | WATTANASAKULPONG, N. and UNGBHAKORN, V. Linear and nonlinear vibration analysis of elastically restrained ends FGM beams with porosities. Aerospace Science and Technology, 32(1), 111–120 (2014) |
| [29] | TANG, Y. and YANG, T. Post-buckling behavior and nonlinear vibration analysis of a fluid-conveying pipe composed of functionally graded material. Composite Structures, 185, 393–400 (2018) |
| [30] | REDDY, R. S., PANDA, S., and GUPTA, A. Nonlinear dynamics of an inclined FG pipe conveying pulsatile hot fluid. International Journal of Non-Linear Mechanics, 118, 103276 (2020) |
| [31] | ZHU, B., XU, Q., LI, M., and LI, Y. Nonlinear free and forced vibrations of porous functionally graded pipes conveying fluid and resting on nonlinear elastic foundation. Composite Structures, 252, 112672 (2020) |
| [32] | ZHEN, Y., GONG, Y., and TANG, Y. Nonlinear vibration analysis of a supercritical fluid-conveying pipe made of functionally graded material with initial curvature. Composite Structures, 268, 113980 (2021) |
| [33] | MAO, X. Y., JING, J., DING, H., and CHEN, L. Q. Dynamics of axially functionally graded pipes conveying fluid. Nonlinear Dynamics, 111(12), 11023–11044 (2023) |
| [34] | CHEN, L. Q. and TANG, Y. Q. Combination and principal parametric resonances of axially accelerating viscoelastic beams: recognition of longitudinally varying tensions. Journal of Sound and Vibration, 330(23), 5598–5614 (2011) |
| [35] | TANG, Y. Q., ZHANG, D. B., and GAO, J. M. Parametric and internal resonance of axially accelerating viscoelastic beams with the recognition of longitudinally varying tensions. Nonlinear Dynamics, 83(1-2), 401–418 (2016) |
| [36] | MAO, X. Y., DING, H., and CHEN, L. Q. Steady-state response of a fluid-conveying pipe with 3:1 internal resonance in supercritical regime. Nonlinear Dynamics, 86(2), 795–809 (2016) |
| [1] | Lele REN, Wei ZHANG, Yufei ZHANG. Inter-well internal resonance analysis of rectangular asymmetric cross-ply bistable composite laminated cantilever shell under transverse foundation excitation [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(8): 1353-1370. |
| [2] | Bo DOU, Hu DING, Xiaoye MAO, Sha WEI, Liqun CHEN. Dynamic modeling of fluid-conveying pipes restrained by a retaining clip [J]. Applied Mathematics and Mechanics (English Edition), 2023, 44(8): 1225-1240. |
| [3] | Shuguang LI, M. I. KHAN, F. ALI, S. S. ABDULLAEV, S. SAADAOUI, HABIBULLAH. Mathematical modeling of mixed convective MHD Falkner-Skan squeezed Sutterby multiphase flow with non-Fourier heat flux theory and porosity [J]. Applied Mathematics and Mechanics (English Edition), 2023, 44(11): 2005-2018. |
| [4] | Xinte WANG, Juan LIU, Biao HU, Bo ZHANG, Huoming SHEN. Wave propagation responses of porous bi-directional functionally graded magneto-electro-elastic nanoshells via nonlocal strain gradient theory [J]. Applied Mathematics and Mechanics (English Edition), 2023, 44(10): 1821-1840. |
| [5] | Rui SONG, S. SAHMANI, B. SAFAEI. Isogeometric nonlocal strain gradient quasi-three-dimensional plate model for thermal postbuckling of porous functionally graded microplates with central cutout with different shapes [J]. Applied Mathematics and Mechanics (English Edition), 2021, 42(6): 771-786. |
| [6] | M. ESMAEILZADEH, M. KADKHODAYAN, S. MOHAMMADI, G. J. TURVEY. Nonlinear dynamic analysis of moving bilayer plates resting on elastic foundations [J]. Applied Mathematics and Mechanics (English Edition), 2020, 41(3): 439-458. |
| [7] | Xun WANG, Chunxia XUE, Haitao LI. Nonlinear primary resonance analysis for a coupled thermo-piezoelectric-mechanical model of piezoelectric rectangular thin plates [J]. Applied Mathematics and Mechanics (English Edition), 2019, 40(8): 1155-1168. |
| [8] | Yunyue CONG, Houjun KANG, Tieding GUO. Analysis of in-plane 1:1:1 internal resonance of a double cable-stayed shallow arch model with cables' external excitations [J]. Applied Mathematics and Mechanics (English Edition), 2019, 40(7): 977-1000. |
| [9] | Yanqing WANG, Chao YE, J. W. ZU. Identifying the temperature effect on the vibrations of functionally graded cylindrical shells with porosities [J]. Applied Mathematics and Mechanics (English Edition), 2018, 39(11): 1587-1604. |
| [10] | A. K. SINGH, A. DAS, A. RAY. Rayleigh-type wave propagation through liquid layer over corrugated substrate [J]. Applied Mathematics and Mechanics (English Edition), 2017, 38(6): 851-866. |
| [11] | Hu DING, Linglu HUANG, Xiaoye MAO, Liqun CHEN. Primary resonance of traveling viscoelastic beam under internal resonance [J]. Applied Mathematics and Mechanics (English Edition), 2017, 38(1): 1-14. |
| [12] | Yizhao WAN, Yuewu LIU, Weiping OUYANG, Guofeng HAN, Wenchao LIU. Numerical investigation of dual-porosity model with transient transfer function based on discrete-fracture model [J]. Applied Mathematics and Mechanics (English Edition), 2016, 37(5): 611-626. |
| [13] | Zhijun LIU, Tangdai XIA, Qingqing ZHENG, Weiyun CHEN. Comparison about parametric effects on wave propagation characteristics [J]. Applied Mathematics and Mechanics (English Edition), 2015, 36(6): 763-776. |
| [14] | Wenan JIANG, Guoce ZHANG, Liqun CHEN. Forced response of quadratic nonlinear oscillator: comparison of various approaches [J]. Applied Mathematics and Mechanics (English Edition), 2015, 36(11): 1403-1416. |
| [15] | Hai YU;Yu-shu CHEN;Qing-jie CAO. Bifurcation analysis for nonlinear multi-degree-of-freedom rotor system with liquid-film lubricated bearings [J]. Applied Mathematics and Mechanics (English Edition), 2013, 34(6): 777-790. |
| Viewed | ||||||
|
Full text |
|
|||||
|
Abstract |
|
|||||


 Email Alert
Email Alert RSS
RSS