Fig. 1

Description of the piezoelectric meta-beam electrical-mechanical system: illustration of the unidirectional ampliflcation electrical element encased in a dashed box (referenced as Fig. 2), where the orientation depictions with the x-, y-, and z-axes signify the length, width, and height, respectively, the piezoelectric patch has a length lp and a height hp, the substrate section has a length ls and a height hs, and all widths are standardized to b (color online)"

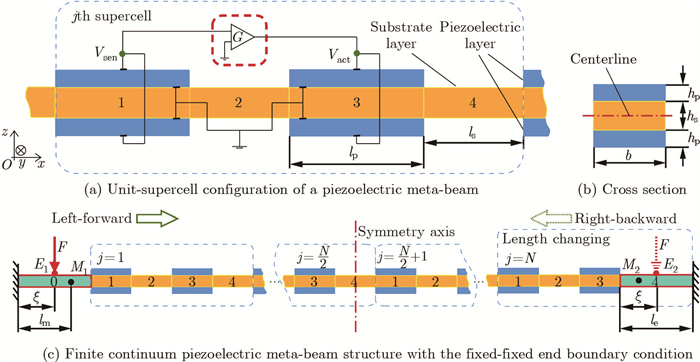
Fig. 2

(a) Schematic representation of unidirectional amplification control circuitry (encapsulated within the dashed framework). (b) Conceptual illustration of phase-reversal amplification electrical component. (c) Conceptual illustration of unidirectional in-phase amplification electrical component (color online)"

Table 1

Geometrical and physical parameters of a piezoelectric meta-beam supercell"

Fig. 3

Validation of analytical methods for solving cantilever meta-beam transmission with varying displacement boundary conditions (color online)"

Table 2

The flrst eight natural frequencies of the cantilever meta-beam under control with G = -10"

Fig. 4

Validation of analytical methods for solving flxed-flxed meta-beam transmission under both forward and backward excitation conditions (color online)"

Fig. 5

Broadband vibration control frequency response diagram with excitation on Point E2 and vibration signal monitoring on Point M1 (color online)"

Fig. 6

Normalized colormaps of maximum vibration displacement responses in a piezoelectric beam subject to difierent excitation positions and control conflgurations (color online)"