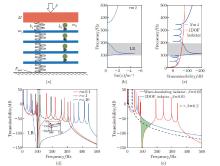
Fig. 1

Properties for the wave-insulating vibration isolator: (a) principal model of the wave-insulating vibration isolator; (b) imaginary part of the wave vector κ; (c) vibration transmissibility for the wave-insulating vibration isolator (in blue) and the 1-degree-of-freedom (1DOF) isolator (in red); (d) influence of the mass ratio r on the bandgap width; (e) comparison of isolation effects between the wave-insulating isolator and the 2-degree-of-freedom (2DOF) isolator (color online)"

Fig. 2

Model of a wave-insulation isolator: (a) the overall structure of the isolator system; (b) one plate-shaped LR unit, consisting primarily of a rectangular plate and four elastic elements; (c) an elastic element structure (color online)"

Fig. 3

An accurate model for the boundary conditions of plate: (a) the relationship between the Bloch boundary and elastic boundary; (b) displacement diagram of the metacell structure (color online)"

Table 1

Dimensionless coefficients λij2 of the free boundary"

Table 2

Dimensionless coefficients λij2 of the simply-supported plate at four-corners"

Fig. 4

Dispersion curves and mode shapes of the plate-shaped LR unit: (a) comparison diagram for the dispersion curve calculation by the analytical method (in red circle) and FEM (in blue dots); (b) the mode shape of the plate when κ=0; (c) the mode shape of the plate when κ=π/L (color online)"

Fig. 5

Dispersion curves of the LR unit: (a) SS mode shape and SS mode bandgap; (b) LR unit and different bandgaps; (c) SA mode shape and SA mode bandgap (color online)"

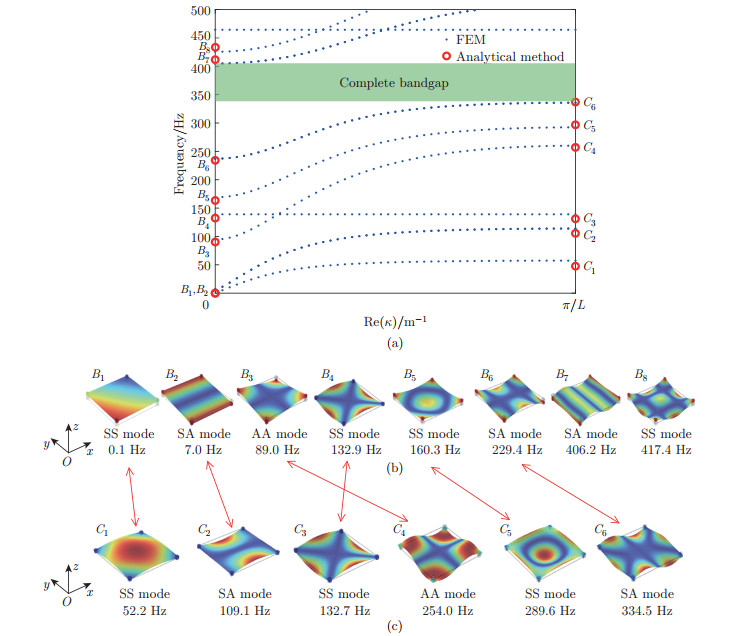
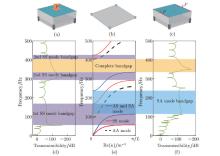
Fig. 6

Vibration isolation characteristics of the wave-insulating isolator: (a) diagram of P applied at the top of the isolated object; (b) schematic diagram of one plate-shaped LR unit used to calculate the bandgap; (c) diagram of F applied at the top of the isolated object; (d) transmissibility under P; (e) dispersion curve of the LR unit; (f) transmissibility under F (color online)"

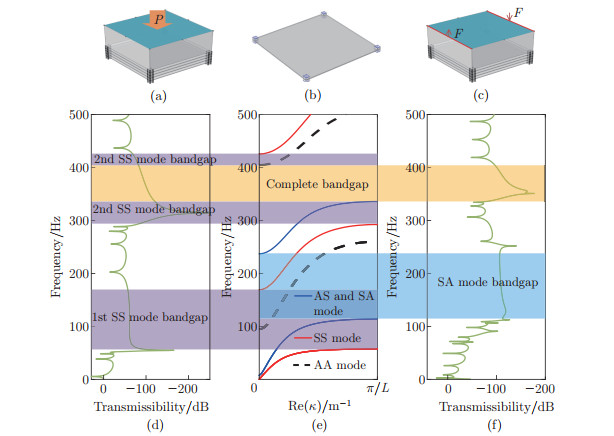
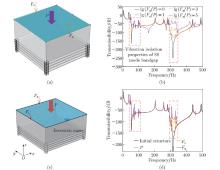
Fig. 7

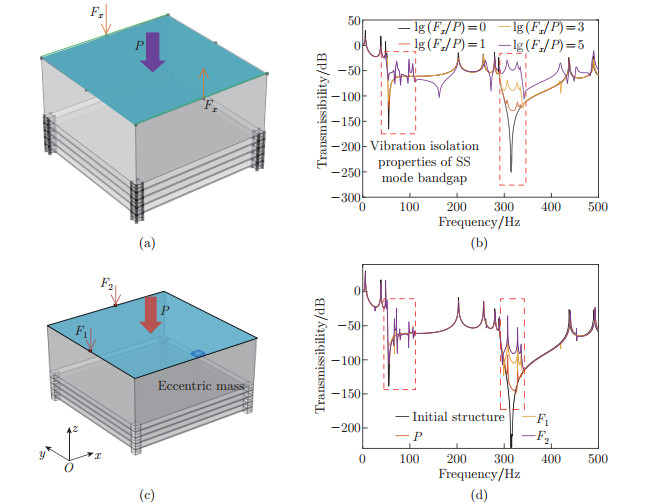
Robustness of the SS mode bandgap under complex excitations and structures: (a) schematic diagram of the eccentric excitation; (b) transmissibility for the SS mode bandgap under the eccentric excitation; (c) schematic diagram of the eccentric structure; (d) transmissibility for the SS mode bandgap under the eccentric structure (color online)"

Fig. 8

Robustness of the SA mode bandgap under complex excitations and structures: (a) schematic diagram of the eccentric structure; (b) transmissibility for the SA mode bandgap under the eccentric structure (color online)"

Fig. 9

Effects of a1 and k1 on the 1st SS mode bandgap: (a) starting and ending frequencies; (b) normalized bandwidth (color online)"

Fig. 10

Effects of a1 and k1 on the transmissibility: (a) constant a1 and varying k1; (b) constant k1 and varying a1 (color online)"

Fig. 11

Transmissibility of damping in the load-bearing elements and plates: (a) damping coefficient c in load-bearing elements; (b) damping coefficient c in plates (color online)"

Fig. 12

Schematic diagram of the experiment: (a) test sample of the wave-insulating isolator; (b) comparative test sample of the conventional isolator with the same spring stiffness; (c) comparative test sample of the conventional isolator at the same resonant frequency (color online)"

Fig. 13

Experimental results of the wave-insulating isolator (in red), simulation computed by the FEM (in blue), and contrast experiments for the same spring stiffness k1 (in green) and resonant frequency (in black) (color online)"

Fig. 14

Schematic diagram and experimental results for asymmetric structures: (a) an isolator under asymmetric structures; (b) results from different acceleration sensors (color online)"