Applied Mathematics and Mechanics (English Edition) ›› 2024, Vol. 45 ›› Issue (11): 1913-1928.doi: https://doi.org/10.1007/s10483-024-3184-7
• Articles • Previous Articles Next Articles
Huaqing JIN1, Haicheng ZHANG1,2,*( ), Ye LU3, Daolin XU1
), Ye LU3, Daolin XU1
Received:2024-06-25
Online:2024-11-03
Published:2024-10-30
Contact:
Haicheng ZHANG
E-mail:zhanghc@hnu.edu.cn
Supported by:2010 MSC Number:
Huaqing JIN, Haicheng ZHANG, Ye LU, Daolin XU. Floating periodic pontoons for broad bandgaps of water waves. Applied Mathematics and Mechanics (English Edition), 2024, 45(11): 1913-1928.

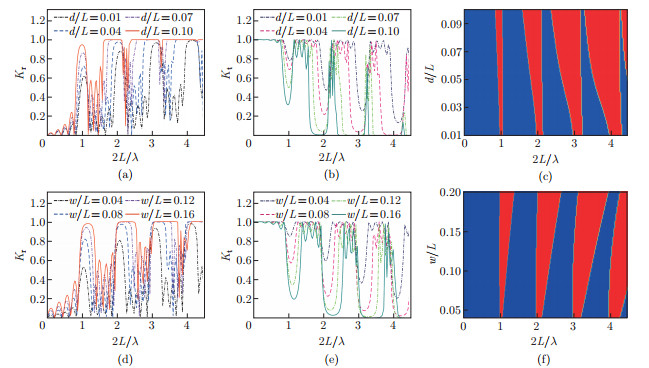
Fig. 10
Effects of the pontoons draft on (a) wave reflection, (b) wave transmission, and (c) bandgaps. Effects of the pontoons width on (d) wave reflection, (e) wave transmission, and (f) bandgaps. The parameters for this case are L=10 m, A/L=0.01, and h/L=2. Pontoon width w/L=0.05 for (a), (b), and (c), and pontoon draft d/L=0.01 for (d), (e), and (f). The wave frequencies range from 0.04 rad/s to 3.7 rad/s (color online)"
| 1 |
WANG,K.,ZHOU,J. X.,TAN,D. G.,LI,Z. Y.,LIN,Q. D., andXU,D. L.A brief review of metamaterials for opening low-frequency band gaps.Applied Mathematics and Mechanics (English Edition),43(7),1125-1144(2022)
doi: 10.1007/s10483-022-2870-9 |
| 2 |
WANG,Z. Y.,MA,Z. Y.,GUO,X. M., andZHANG,D. S.A new tunable elastic metamaterial structure for manipulating band gaps/wave propagation.Applied Mathematics and Mechanics (English Edition),42(11),1543-1554(2021)
doi: 10.1007/s10483-021-2787-8 |
| 3 |
YANG,F.,MA,Z. Y., andGUO,X. M.Bandgap characteristics of the two-dimensional missing rib lattice structure.Applied Mathematics and Mechanics (English Edition),43(11),1631-1640(2022)
doi: 10.1007/s10483-022-2923-6 |
| 4 | HEATHERSHAW,A. D.Seabed-wave resonance and sand bar growth.nature,296(5855),343-345(1982) |
| 5 |
WU,Z. R.,CHENG,Y. L.,WANG,S. L., andLU,Y. K.Effects of varying bottom on nonlinear surface waves.Applied Mathematics and Mechanics (English Edition),27(3),409-416(2006)
doi: 10.1007/s10483-006-0318-y |
| 6 | KIRBY, J. T. and ANTON, J. P. Bragg reflection of waves by artificial bars. Proceedings of the 22nd Intel-national Conference of Coastal Engineering, New York, 757–768 (1990) |
| 7 | CHANG,H. K., andLIOU,J. C.Long wave reflection from submerged trapezoidal breakwaters.Ocean Engineering,34(1),185-191(2007) |
| 8 | ZORKANI,M., andHEINRICHS,J.Bragg reflection and localization of hydrodynamic waves in a channel of variable width: theory and experiment.Europhysics Letters,14(3),243-248(1991) |
| 9 | HSU,T. W.,LIN,J. F.,HSIAO,S. C.,OU,S. H.,BABANIN,A. V., andWU,Y. T.Wave reflection and vortex evolution in Bragg scattering in real fluids.Ocean Engineering,88,508-519(2014) |
| 10 | ZENG,H.,QIN,B., andZHANG,J.Optimal collocation of Bragg breakwaters with rectangular bars on sloping seabed for Bragg resonant reflection by long waves.Ocean Engineering,130,156-165(2017) |
| 11 | NING,D.,ZHANG,S.,CHEN,L.,LIU,H. W., andTENG,B.Nonlinear Bragg scattering of surface waves over a two-dimensional periodic structure.Journal of Fluid Mechanics,946,A25(2022) |
| 12 | TORRES,M.,ADRADOS,J. P., andDE ESPINOSA,F. R. M.Visualization of Bloch waves and domain walls.nature,398(6723),114-115(1999) |
| 13 | CHOU,T.Band structure of surface flexural-gravity waves along periodic interfaces.Journal of Fluid Mechanics,369,333-350(1998) |
| 14 | AN,Z., andYE,Z.Band gaps and localization of water waves over one-dimensional topographical bottoms.Applied Physics Letters,84(15),2952-2954(2004) |
| 15 | HUANG,M. J.,KOU,C. H., andYE,Z.Gravity waves over topographical bottoms: comparison with experiment.Physical Review E,71(1),011201(2005) |
| 16 | PORTER,R., andPORTER,D.Scattered and free waves over periodic beds.Journal of Fluid Mechanics,483,129-163(2003) |
| 17 | LIU,H. W.Band gaps for Bloch waves over an infinite array of trapezoidal bars and triangular bars in shallow water.Ocean Engineering,130,72-82(2017) |
| 18 | LIU,H. W.,LIU,Y., andLIN,P.Bloch band gap of shallow-water waves over infinite arrays of parabolic bars and rectified cosinoidal bars and Bragg resonance over finite arrays of bars.Ocean Engineering,188,106235(2019) |
| 19 | CUI,J.,CONG,L., andTENG,B.An eigenvalue method based on the integral equation with a Rankine source for the band structure of water surface waves over periodic scatterers.Ocean Engineering,283,114911(2023) |
| 20 | JIN,H.,ZHANG,H., andXU,D.Array buoys with nonlinear stiffness enhance low-frequency wave attenuation and energy capture.Physics of Fluids,34(12),127106(2022) |
| 21 | LIU,C., andYANG,W.Theoretical analysis and numerical study on a flexible piezoelectric wave energy converter.International Journal of Hydromechatronics,5(4),292-310(2022) |
| 22 |
ZHANG,X. T.,ZHANG,H. C.,ZHOU,X., andSUN,Z.Recent advances in wave energy converters based on nonlinear stiffness mechanism.Applied Mathematics and Mechanics (English Edition),43(7),1081-1108(2022)
doi: 10.1007/s10483-022-2864-6 |
| 23 | JIN,H.,ZHANG,H.,XU,D.,LIU,C., andXU,S.Analytical investigation on wave attenuation performance of a floating breakwater with nonlinear stiffness.Ocean Engineering,243,110160(2022) |
| 24 | LINTON,C. M.Water waves over arrays of horizontal cylinders: band gaps and Bragg resonance.Journal of Fluid Mechanics,670,504-526(2011) |
| 25 | DAI,J.,WANG,C. M.,UTSUNOMIYA,T., andDUAN,W.Review of recent research and developments on floating breakwaters.Ocean Engineering,158,132-151(2018) |
| 26 | FALNES,J.Ocean Waves and Oscillating Systems: Linear Interactions Including Wave-Energy Extraction,Cambridge University Press,Cambridge(2002) |
| 27 | ZHENG,S., andZHANG,Y.Wave diffraction and radiation by multiple rectangular floaters.Journal of Hydraulic Research,54(1),102-115(2016) |
| 28 | ZHENG,Y.,YOU,Y., andSHEN,Y.On the radiation and diffraction of water waves by a rectangular buoy.Ocean Engineering,31(8-9),1063-1082(2004) |
| 29 | OUYANG,H. T.,CHEN,K. H., andTSAI,C. M.Investigation on bragg reflection of surface water waves induced by a train of fixed floating pontoon breakwaters.International Journal of Naval Architecture and Ocean Engineering,7(6),951-963(2015) |
| 30 | KAR,P.,SAHOO,T., andMEYLAN,M. H.Bragg scattering of long waves by an array of floating flexible plates in the presence of multiple submerged trenches.Physics of Fluids,32(9),096603(2020) |
| 31 | LIU,H. W.An approximate law of class I Bragg resonance of linear shallow-water waves excited by five types of artificial bars.Ocean Engineering,267,113245(2023) |
| 32 | LING,Z.,LI,Y., andZHANG,Y.Investigation of Bragg resonant reflection of linear and nonlinear water waves by multiple fixed floating structures based on viscous meshfree method.Ocean Engineering,278,114270(2023) |
| 33 | DING,Y.,LIU,H. W., andLIN,P.Quantitative expression of the modified Bragg's law for Bragg resonances of water waves excited by five types of artificial bars.Physics of Fluids,36(4),047130(2024) |
| 34 | LIU,H. W.,ZENG,H. D., andHUANG,H. D.Bragg resonant reflection of surface waves from deep water to shallow water by a finite array of trapezoidal bars.Applied Ocean Research,94,101976(2020) |
| 35 | DONG,H. W.,ZHAO,S. D.,WANG,Y. S., andZHANG,C. Z.Topology optimization of anisotropic broadband double-negative elastic metamaterials.Journal of the Mechanics and Physics of Solids,105,54-80(2017) |
| [1] | Wei WEI, Feng GUAN, Xin FANG. A low-frequency and broadband wave-insulating vibration isolator based on plate-shaped metastructures [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(7): 1171-1188. |
| [2] | Changqi CAI, Chenjie ZHU, Fengyi ZHANG, Jiaojiao SUN, Kai WANG, Bo YAN, Jiaxi ZHOU. Modeling and analysis of gradient metamaterials for broad fusion bandgaps [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(7): 1155-1170. |
| [3] | Xingjian DONG, Shuo WANG, Anshuai WANG, Liang WANG, Zhaozhan ZHANG, Yuanhao TIE, Qingyu LIN, Yongtao SUN. Low-frequency bandgap and vibration suppression mechanism of a novel square hierarchical honeycomb metamaterial [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(10): 1841-1856. |
| [4] | Yunping ZHAO, Xiuhui HOU, Kai ZHANG, Zichen DENG. Symplectic analysis for regulating wave propagation in a one-dimensional nonlinear graded metamaterial [J]. Applied Mathematics and Mechanics (English Edition), 2023, 44(5): 745-758. |
| [5] | Feiyang HE, Zhiyu SHI, Denghui QIAN, Y. K. LU, Yujia XIANG, Xuelei FENG. Flexural wave bandgap properties of phononic crystal beams with interval parameters [J]. Applied Mathematics and Mechanics (English Edition), 2023, 44(2): 173-188. |
| [6] | Xiaosheng CAI, Nenghui ZHANG, Hanlin LIU. Thickness dependence of viscoelastic stress relaxation of laminated microbeams due to mismatch strain [J]. Applied Mathematics and Mechanics (English Edition), 2022, 43(4): 467-478. |
| [7] | Fan YANG, Zhaoyang MA, Xingming GUO. Bandgap characteristics of the two-dimensional missing rib lattice structure [J]. Applied Mathematics and Mechanics (English Edition), 2022, 43(11): 1631-1640. |
| [8] | Chenxu QIANG, Yuxin HAO, Wei ZHANG, Jinqiang LI, Shaowu YANG, Yuteng CAO. Bandgaps and vibration isolation of local resonance sandwich-like plate with simply supported overhanging beam [J]. Applied Mathematics and Mechanics (English Edition), 2021, 42(11): 1555-1570. |
| [9] | Xinchun SHANG, Jiaxuan LIU, Xuhua GAO, Weiyao ZHU. Analysis on nonlinear effect of unsteady percolation in the inhomogeneous shale gas reservoir [J]. Applied Mathematics and Mechanics (English Edition), 2020, 41(1): 105-122. |
| [10] | R. ANSARI, T. POURASHRAF, R. GHOLAMI, H. ROUHI. Analytical solution approach for nonlinear buckling and postbuckling analysis of cylindrical nanoshells based on surface elasticity theory [J]. Applied Mathematics and Mechanics (English Edition), 2016, 37(7): 903-918. |
| [11] | K. REGER;R. A. VAN GORDER. Lane-Emden equations of second kind modelling thermal explosion in infinite cylinder and sphere [J]. Applied Mathematics and Mechanics (English Edition), 2013, 34(12): 1439-1452. |
| [12] | LUO Lin;XIANG Yu;WANG Qi-Zhi. Stress concentration factor expression for tension strip with eccentric elliptical hole [J]. Applied Mathematics and Mechanics (English Edition), 2012, 33(1): 117-128. |
| [13] | PENG Jian-She;YANG Jie;YUAN Yu-Quan;LUO Guang-Bing. A convolution-type semi-analytic DQ approach to transient response of rectangular plates [J]. Applied Mathematics and Mechanics (English Edition), 2009, 30(9): 1143-1151. |
| [14] | GONG Wen-hui;XIE Hong-yang;WANG Yuan-han. SEMI-ANALYTICAL AND SEMI-NUMERICAL METHOD FOR DYNAMIC ANALYSIS OF FOUNDATION [J]. Applied Mathematics and Mechanics (English Edition), 2006, 27(5): 607-615 . |
| [15] | HUANG Yan;LEI Yong-jun;SHEN Hui-jun. FREE VIBRATION OF ANISOTROPIC RECTANGULAR PLATES BY GENERAL ANALYTICAL METHOD [J]. Applied Mathematics and Mechanics (English Edition), 2006, 27(4): 461-467 . |
| Viewed | ||||||
|
Full text |
|
|||||
|
Abstract |
|
|||||


 Email Alert
Email Alert RSS
RSS