Applied Mathematics and Mechanics (English Edition) ›› 2024, Vol. 45 ›› Issue (12): 2055-2074.doi: https://doi.org/10.1007/s10483-024-3194-9
• Articles • Next Articles
Jianguo CUI1, Tianzhi YANG2, Wenju HAN3, Liang LI1, Muqing NIU4, Liqun CHEN5,6,*( )
)
Received:2024-07-30
Online:2024-12-01
Published:2024-11-30
Contact:
Liqun CHEN
E-mail:lqchen@shu.edu.cn
Supported by:2010 MSC Number:
Jianguo CUI, Tianzhi YANG, Wenju HAN, Liang LI, Muqing NIU, Liqun CHEN. Tunable topological interface states via a parametric system in composite lattices with/without symmetric elements. Applied Mathematics and Mechanics (English Edition), 2024, 45(12): 2055-2074.

Fig. 2
The dispersion relation of periodic lattices, where $ f_{\rm Ⅰ} $ to $ f_{\rm Ⅳ} $ are the boundaries of the acoustic and optical bands, and the parametric values are assumed to be $ \alpha_1=1.5 $, $ \alpha_2=0.5 $, $ \mu_1=1.5 $, $ \mu_2=0.5 $, $ \omega_{\rm e}=10 $, and $ \beta=0.1 $ (color online)"


Fig. 3
Band transition in a 1D lattice system: (a) the band structures for different stiffnesses of connection springs $ \alpha_1 $ and masses of the element mass $ \mu_1 $, (b) the band transition at $ \mu_1=\mu_2 =1 $ with the increasing dimensionless stiffness $ \alpha_1 $, (c) the band transition at $ \alpha_1=\alpha_2 =1 $ with the increasing dimensionless stiffness $ \mu_1 $, and (d) the band transition at $ \mu_1 =1.5 $ and $ \mu_2=0.5 $ with the increasing dimensionless stiffness $ \alpha_1 $ (color online)"


Fig. 4
Trend of the transition point frequencies, where the $ x $-axis represents the parametric excitation frequency, the $ y $-axis represents the transition frequency, and the red points indicate the transition point frequencies corresponding to the parametric excitation frequencies $ \omega_{\rm e} $ of 0, 10, 20, and 30, respectively (color online)"


Fig. 5
(a) The frequency response function showing the interface mode within the band gap, when $ \alpha_1=1.5 $, $ \alpha_2=0.5 $, $ \mu_1=1 $, $ \mu_2=1 $, $ \beta=0.1 $, and $ \omega_{\rm e}=20 $. (b) The displacement distribution along the entire topological combination lattices, where the $ x $-axis represents the number of elements based on the global metamaterial structure, and the $ y $-axis denotes the amplitudes of element responses (color online)"


Fig. 6
The frequency response function showing the interface mode within the band gap with varying parametric excitations for Case 1, where the solid lines represent the frequency responses for the CL, the dotted lines denote the frequency responses for the SL, the yellow stars denote the frequency and response of the topological transition points, the wax yellow dotted dash lines denote the transition points of the analytical predictions, and different colored lines denote the frequency responses at the excitation frequencies $ \omega_{\rm e} =0, 10, 20, 30 $, respectively (color online)"


Fig. 7
The frequency response function showing the interface mode within the band gap with varying parametric excitations for Case 2, where the wax yellow dotted-dash lines denote the transition points of the analytical prediction, and different colored lines denote the frequency responses at the excitation frequencies $ \omega_{\rm e} =0 $ and 30, respectively (color online)"


Fig. 8
The frequency response function showing the interface mode within the band gap with varying parametric excitations for Case 3, where the wax yellow dotted-dash lines denote the transition points of the analytical prediction, and different colored lines denote the frequency responses at the excitation frequencies $ \omega_{\rm e}=0 $ and 30, respectively (color online)"


Fig. 9
The frequency response function showing the interface mode within the band gap with varying parametric excitations for Case 4, where the wax yellow dotted-dash lines denote the transition points of the analytical prediction, and different colored lines denote the frequency responses at the excitation frequencies $ \omega_{\rm e}=0 $ and 30, respectively (color online)"


Fig. 10
The frequency response function showing the interface mode within the band gap with varying middle masses and parametric excitations for Case 1, where the wax yellow dotted-dash line denotes the transition point of the analytical predictions, different symbols denote the variable middle mass with $ \mu_{\rm c}=0.5 $, 1, and 1.5, respectively, and different colored lines denote the frequency responses at the excitation frequencies $ \omega_{\rm e}=0 $ and 20, respectively (color online)"


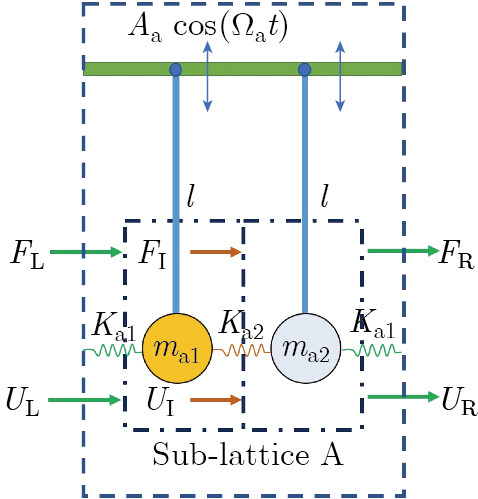
Fig. B1
The schematic of internal force and displacement transfer from left to right ends of the unit cell, where $ F_{\rm L} $ and $ U_{\rm L} $ are the force and displacement on the left, respectively, $ F_{\rm I} $ and $ U_{\rm I} $ are the force and displacement on the middle, respectively, and $ F_{\rm R} $ and $ U_{\rm R} $ are the force and displacement on the right, respectively (color online)"
| 1 | NASSAR, H., YOUSEFZADEH, B., FLEURY, R., RUZZENE, M., ALU, A., DARAIO, C., NORRIS, A. N., HUANG, G. L., and HABERMAN, M. R. Nonreciprocity in acoustic and elastic materials. Nature Reviews Materials, 5 (9), 667- 685 (2020) |
| 2 | JIN, K. H., JIANG, W., SETHI, G., and LIU, F. Topological quantum devices: a review. Nanoscale, 15 (31), 12787- 12817 (2023) |
| 3 | CHEN, H., NASSAR, H., and HUANG, G. A study of topological effects in 1D and 2D mechanical lattices. Journal of the Mechanics and Physics of Solids, 117, 22- 36 (2018) |
| 4 | PERI, V., SONG, Z. D., SERRA-GARCIA, M., ENGELER, P., QUEIROZ, R., HUANG, X. Q., DENG, W. Y., LIU, Z. Y., BERNEVIG, B. A., and HUBER, S. D. Experimental characterization of fragile topology in an acoustic metamaterial. Science, 367 (6479), 797- 800 (2020) |
| 5 | PAL, R. K., and RUZZENE, M. Edge waves in plates with resonators: an elastic analogue of the quantum valley Hall effect. New Journal of Physics, 19, 025001 (2017) |
| 6 | FAN, L., CHEN, Y. F., ZHU, J., and SU, Z. Q. Multi-band topological valley modes of flexural waves in micro-perforated phononic plates. International Journal of Mechanical Sciences, 266, 108916 (2023) |
| 7 | PAL, R. K., VILA, J., LEAMY, M., and RUZZENE, M. Amplitude-dependent topological edge states in nonlinear phononic lattices. Physical Review E, 97 (3), 032209 (2018) |
| 8 | GAO, N., QU, S., SI, L., WANG, J., and CHEN, W. Broadband topological valley transport of elastic wave in reconfigurable phononic crystal plate. Applied Physics Letters, 118 (6), 063502 (2021) |
| 9 | HU, G. B., LAN, C., TANG, L. H., and YANG, Y. W. Local resonator stimulated polarization transition in metamaterials and the formation of topological interface states. Mechanical Systems and Signal Processing, 165, 108388 (2022) |
| 10 | LI, P., HU, W. P., PENG, P., ZHU, X. F., and ZHAO, D. G. Elastic topological interface states induced by incident angle. International Journal of Mechanical Sciences, 225, 107359 (2022) |
| 11 | GE, Y., SHI, B. J., XIA, J. P., SUN, H. X., YUAN, S. Q., XUE, H. R., and ZHANG, B. L. Programmable dual-band acoustic topological insulator with dynamically movable interface states. Applied Physics Reviews, 10 (3), 031403 (2023) |
| 12 | MA, G. C., XIAO, M., and CHAN, C. T. Topological phases in acoustic and mechanical systems. Nature Reviews Physics, 1 (4), 281- 294 (2019) |
| 13 | HAN, N., LIU, J. L., GAO, Y., ZHOU, K. Y., and LIU, S. T. Topological phase transitions and Weyl semimetal phases in chiral photonic metamaterials. New Journal of Physics, 24 (5), 053052 (2022) |
| 14 | BERRY, M. V., and WILKINSON, M. Diabolical points in the spectra of triangles. Proceedings of the Royal Society London, 392 (1802), 15- 43 (1984) |
| 15 | WANG, J. W., VALLIGATLA, S., LI, S. L., YIN, Y., SCHWARZ, L., MEDINA-SANCHEZ, M., BAUNACK, S., LEE, C. H., THOMALE, R., FOMIN, V., MA, L. B., and SCHMIDT, O. Experimental observation of Berry phases in optical Mobius-strip microcavities. Nature Photonics, 17 (1), 120- 125 (2023) |
| 16 | XIAO, M., MA, G. C., YANG, Z. Y., SHENG, P., ZHANG, Z. Q., and CHAN, C. T. Geometric phase and band inversion in periodic acoustic systems. Nature Physics, 11 (3), 240- 244 (2015) |
| 17 | COHEN, E., LAROCQUE, H., BOUCHARD, F., NEJADSATTARI, F., GEFEN, Y., and KARIMI, E. Geometric phase from Aharonov-Bohm to Pancharatnam-Berry and beyond. Nature Reviews Physics, 1 (7), 437- 449 (2019) |
| 18 | ZAK, J. Berry's phase for energy bands in solids. Physical Review Letters, 62, 2747- 2750 (1989) |
| 19 | LIU, C., WANG, H. R., and ONG, H. C. Determination of the Zak phase of one-dimensional diffractive systems with inversion symmetry via radiation in Fourier space. Physical Review B, 108 (3), 035403 (2023) |
| 20 | TANG, G. J., HE, X. T., SHI, F. L., LIU, J. W., CHEN, X. D., and DONG, J. W. Topological photonic crystals: physics, designs, and applications. Laser & Photonics Reviews, 16 (4), 2100300 (2022) |
| 21 | LONGHI, S. Probing one-dimensional topological phases in waveguide lattices with broken chiral symmetry. Optics Letters, 43 (19), 4639- 4642 (2018) |
| 22 | ZHANG, X. Z., and SONG, Z. Partial topological Zak phase and dynamical confinement in a non-Hermitian bipartite system. Physical Review A, 99 (1), 012113 (2019) |
| 23 | ZHU, W. W., DING, Y. Q., REN, J., SUN, Y., LI, Y. H., JIANG, H. T., and CHEN, H. Zak phase and band inversion in dimerized one-dimensional locally resonant metamaterials. Physical Review B, 97 (19), 195307 (2018) |
| 24 | PENG, Y. G., GENG, Z. G., and ZHU, X. F. Topologically protected bound states in one-dimensional Floquet acoustic waveguide systems. Journal of Applied Physics, 123 (9), 091716 (2018) |
| 25 | LIU, T., FAN, Y. X., ZHANG, J. Y., SU, Y., and TAO, Z. Y. Interface states of dipole-like distributions in a quasi-periodic acoustic waveguide. Applied Acoustics, 181, 108174 (2021) |
| 26 | ZHAO, D. G., XIAO, M., LING, C. W., CHAN, C. T., and FUNG, K. H. Topological interface modes in local resonant acoustic systems. Physical Review B, 98 (1), 014110 (2018) |
| 27 | LYU, X. F., LI, H. Z., MA, Z. S., DING, Q., YANG, T. Z., CHEN, L. Q., and ZUR, K. K. Numerical and experimental evidence of topological interface state in a periodic acoustic black hole. Journal of Sound and Vibration, 514, 116432 (2021) |
| 28 | WANG, H. F., LIU, D. Y., FANG, W. B., LIN, S. Q., LIU, Y. J., and LIANG, Y. J. Tunable topological interface states in one-dimensional extended granular crystals. International Journal of Mechanical Sciences, 176, 105549 (2020) |
| 29 | LIU, Y. J., FANG, W. B., LIANG, Y. J., LIU, D. Z., and HAN, Q. Tuning of subwavelength topological interface states in locally resonant metastructures with shunted piezoelectric patches. Journal of Applied Physics, 129 (24), 245112 (2021) |
| 30 | LIU, Y. J., WANG, H. F., FANG, W. B., HAN, Q., LIU, D. Z., and LIANG, Y. J. Tunable control of subwavelength topological interface modes in locally resonance piezoelectric metamaterials. Composite Structures, 276, 114541 (2021) |
| 31 | CAJIC, M., CHRISTENSEN, J., and ADHIKARI, S. Tuning of topological interface modes in an elastic beam array system with inerters. International Journal of Mechanical Sciences, 205, 106573 (2021) |
| 32 | WANG, S., and WANG, Y. Z. Active control on topological interface states of elastic wave metamaterials with double coupled chains. Journal of the Acoustical Society of America, 154 (4), 2440- 2452 (2023) |
| 33 | HUO, S. Y., YAO, L. C., HSIEH, K. H., FU, C. M., CHIU, S. C., GONG, X. C., and DENG, J. Tunable topological interface states and resonance states of surface waves based on the shape memory alloy. Chinese Physics B, 32 (3), 034303 (2023) |
| 34 | CAJIC, M., KARLICIC, D., CHRISTENSEN, J., and ADHIKARI, S. Tunable topological interface states in one-dimensional inerter-based locally resonant lattices with damping. Journal of Sound and Vibration, 542, 117326 (2023) |
| 35 | ZHANG, S. Z., and BIAN, X. H. Magneto-mechanical-thermal coupling tunability of the topological interface state of longitudinal waves in magnetostrictive phononic crystal beams. Mechanical Systems and Signal Processing, 212, 111286 (2024) |
| 36 |
SONG, Y. Y., CHEN, L. Q., and YANG, T. Z. Geometrically nonlinear inerter for vibration suppression. Applied Mathematics and Mechanics (English Edition), 44 (11), 1871- 1886 (2023)
doi: 10.1007/s10483-023-3051-6 |
| 37 | CHEN, H. Y., MAO, X. Y., DING, H., and CHEN, L. Q. Elimination of multimode resonances of composite plate by inertial nonlinear energy sinks. Mechanical Systems and Signal Processing, 135, 106383 (2020) |
| 38 | YANG, T. Z., DANG, W. H., and CHEN, L. Q. Two-dimensional inerter-enhanced nonlinear energy sink. Nonlinear Dynamics, 112 (1), 379- 401 (2024) |
| 39 | SHARMA, S., KIRAN, R., AZAD, P., and VAISH, R. A review of piezoelectric energy harvesting tiles: available designs and future perspective. Energy Conversion and Management, 254, 115272 (2022) |
| 40 | YANG, T., ZHOU, S. X., FANG, S. T., QIN, W. Y., and INMAN, D. J. Nonlinear vibration energy harvesting and vibration suppression technologies: designs, analysis, and applications. Applied Physics Reviews, 8 (3), 031317 (2021) |
| 41 |
ZHAO, L., LU, Z. Q., DING, H., and CHEN, L. Q. A viscoelastic metamaterial beam for integrated vibration isolation and energy harvesting. Applied Mathematics and Mechanics (English Edition), 45 (7), 1243- 1260 (2024)
doi: 10.1007/s10483-024-3159-7 |
| 42 | ZANGENEH-NEJAD, F., ALU, A., and FLEURY, R. Topological wave insulators: a review. Comptes Rendus Physique, 21 (4-5), 467- 499 (2020) |
| 43 | HUANG, H. B., CHEN, J. J., and HUO, S. Y. Recent advances in topological elastic metamaterials. Journal of Physics-Condensed Matter, 33 (50), 503002 (2021) |
| 44 | YANG, X. D., CUI, Q. D., QIAN, Y. J., ZHANG, W., and LIM, C. W. Modulating band gap structure by parametric excitations. Journal of Applied Mechanics-Transactions of the ASME, 85 (6), 061012 (2018) |
| 45 | CUI, J. G., YANG, T. Z., NIU, M. Q., and CHEN, L. Q. Tunable roton-like dispersion relation with parametric excitations. Journal of Applied Mechanics-Transactions of the ASME, 89 (11), 111005 (2022) |
| 46 | HASAN, M. Z., and KANE, C. L. Colloquium: topological insulators. Reviews of Modern Physics, 82 (4), 3045- 3067 (2010) |
| 47 | BERNEVIG, B. A., HUGHES, T. L., and ZHANG, S. C. Quantum spin hall effect and topological phase transition in HgTe quantum wells. Science, 314 (5806), 1757- 1761 (2006) |
| 48 |
CAO, D. X., WANG, J. R., GUO, X. Y., LAI, S. K., and SHEN, Y. J. Recent advancement of flow-induced piezoelectric vibration energy harvesting techniques: principles, structures, and nonlinear designs. Applied Mathematics and Mechanics (English Edition), 43 (7), 959- 978 (2022)
doi: 10.1007/s10483-022-2867-7 |
| 49 |
YANG, J. H., ZHANG, W. X., and YANG, X. D. Integrated device for multiscale series vibration reduction and energy harvesting. Applied Mathematics and Mechanics (English Edition), 44 (12), 2227- 2242 (2023)
doi: 10.1007/s10483-023-3063-8 |
| [1] | Shuo WANG, Anshuai WANG, Yansen WU, Xiaofeng LI, Yongtao SUN, Zhaozhan ZHANG, Qian DING, G. D. AYALEW, Yunxiang MA, Qingyu LIN. Ultra-wide band gap and wave attenuation mechanism of a novel star-shaped chiral metamaterial [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(7): 1261-1278. |
| [2] | Long ZHAO, Zeqi LU, Hu DING, Liqun CHEN. A viscoelastic metamaterial beam for integrated vibration isolation and energy harvesting [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(7): 1243-1260. |
| [3] | Zhou HU, Zhibo WEI, Yan CHEN, Rui ZHU. Reconfigurable mechanism-based metamaterials for ternary-coded elastic wave polarizers and programmable refraction control [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(7): 1225-1242. |
| [4] | Xingzhong WANG, Shiteng RUI, Shaokun YANG, Weiquan ZHANG, Fuyin MA. A low-frequency pure metal metamaterial absorber with continuously tunable stiffness [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(7): 1209-1224. |
| [5] | Wei WEI, Feng GUAN, Xin FANG. A low-frequency and broadband wave-insulating vibration isolator based on plate-shaped metastructures [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(7): 1171-1188. |
| [6] | Changqi CAI, Chenjie ZHU, Fengyi ZHANG, Jiaojiao SUN, Kai WANG, Bo YAN, Jiaxi ZHOU. Modeling and analysis of gradient metamaterials for broad fusion bandgaps [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(7): 1155-1170. |
| [7] | Yuxin YAO, Yuansheng MA, Fang HONG, Kai ZHANG, Tingting WANG, Haijun PENG, Zichen DENG. On Klein tunneling of low-frequency elastic waves in hexagonal topological plates [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(7): 1139-1154. |
| [8] | Chao WANG, Honggang ZHAO, Yang WANG, Jie ZHONG, Dianlong YU, Jihong WEN. Topology optimization of chiral metamaterials with application to underwater sound insulation [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(7): 1119-1138. |
| [9] | Yabin JING, Lifeng WANG, Yuqiang GAO. Mass-spring model for elastic wave propagation in multilayered van der Waals metamaterials [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(7): 1107-1118. |
| [10] | M. SAFI, M. VAKILIFARD, M.J. MAHMOODI. Frequency-dependent viscoelasticity effects on the wave attenuation performance of multi-layered periodic foundations [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(3): 407-424. |
| [11] | Yu ZHANG, Daming NIE, Xuyao MAO, Li LI. A thermodynamics-consistent spatiotemporally-nonlocal model for microstructure-dependent heat conduction [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(11): 1929-1948. |
| [12] | Jinhui LIU, Yu XUE, Zhihong GAO, A. O. KRUSHYNSKA, Jinqiang LI. Actively tunable sandwich acoustic metamaterials with magnetorheological elastomers [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(11): 1875-1894. |
| [13] | Xingjian DONG, Shuo WANG, Anshuai WANG, Liang WANG, Zhaozhan ZHANG, Yuanhao TIE, Qingyu LIN, Yongtao SUN. Low-frequency bandgap and vibration suppression mechanism of a novel square hierarchical honeycomb metamaterial [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(10): 1841-1856. |
| [14] | Donghai HAN, Qi JIA, Yuanyu GAO, Qiduo JIN, Xin FANG, Jihong WEN, Dianlong YU. Local resonance metamaterial-based integrated design for suppressing longitudinal and transverse waves in fluid-conveying pipes [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(10): 1821-1840. |
| [15] | Jianing LIU, Jinqiang LI, Ying WU. Bandgap adjustment of a sandwich-like acoustic metamaterial plate with a frequency-displacement feedback control method [J]. Applied Mathematics and Mechanics (English Edition), 2024, 45(10): 1807-1820. |
| Viewed | ||||||
|
Full text |
|
|||||
|
Abstract |
|
|||||


 Email Alert
Email Alert RSS
RSS